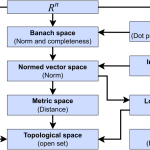
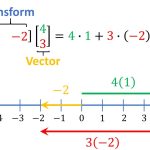
So far our examples of function spaces have been metric spaces (Section 1.3.1). Such spaces are not necessarily associated with the concept of a vector space. However, normed spaces (i.e., spaces with norms defined on them) are always associated with vector spaces. So, before we can define a norm, we need to recall the general definition of a vector space.
The following definition invokes the concept of a field of numbers. This concept arises in abstract algebra and number theory [e.g., 2, 3], a subject we wish to avoid considering here. It is enough for the reader to know that R and C are fields under the usual real and complex arithmetic operations. These are really the only fields that we shall work with. We remark, largely in passing, that rational numbers (set denoted Q) are also a field under the usual arithmetic operations.
Definition 1.2: Vector Space A vector space (linear space) over a field K is a nonempty set X of elements x, y, z, . . . called vectors together with two algebraic operations. These operations are vector addition, and the multiplication of vectors by scalars that are elements of K. The following axioms must be satisfied: