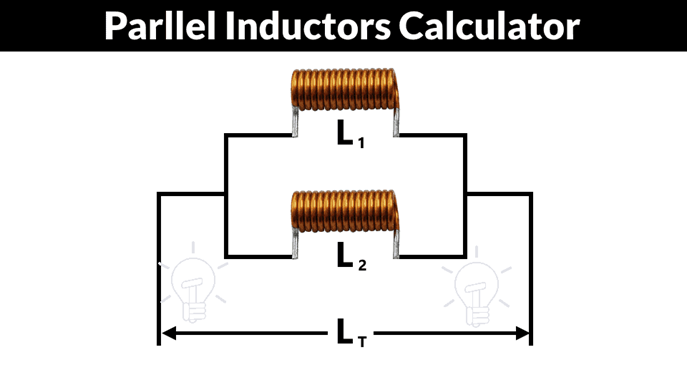
Let us observe what happens, when few resistors are connected in Parallel. Let us consider three resistors with different values, as shown in the figure below.

Inductance
The total inductance of a circuit having Parallel resistors is calculated differently from the series inductor network method. Here, the reciprocal (1/R) value of individual inductances are added with the inverse of algebraic sum to get the total inductance value.
Total inductance value of the network is
1LT=1L1+1L2+1L31LT=1L1+1L2+1L3
Where L1 is the inductance of 1st inductor, L2 is the inductance of 2ndinductor and L3 is the inductance of 3rd inductor in the above network.
From the method we have for calculating parallel inductance, we can derive a simple equation for two-inductor parallel network. It is
LT=L1×L2L1+L2LT=L1×L2L1+L2
Voltage
The total voltage that appears across a Parallel inductors network is same as the voltage drops at each individual inductances.
The Voltage that appears across the circuit
V=V1=V2=V3V=V1=V2=V3
Where V1 is the voltage drop across 1st inductor, V2 is the voltage drop across 2nd inductor and V3 is the voltage drop across 3rd inductor in the above network. Hence the voltage is same at all the points of a parallel inductor network.
Current
The total amount of current entering a Parallel inductive network is the sum of all individual currents flowing in all the Parallel branches. The inductance value of each branch determines the value of current that flows through it.
The total Current through the network is
I=I1+I2+I3I=I1+I2+I3
Where I1 is the current through the 1st inductor, I2 is the current through the 2nd inductor and I3 is the current through the 3rd inductor in the above network.
Hence the sum of individual currents in different branches obtain the total current in a parallel network.